The primorial numbers emerge from the fractions that Emily calculates. The primorials form an integer sequence which is created by multiplying successive prime numbers. The first in the sequence is the first prime number: The second in the sequence is: The third is: Then: And so on. The numbers get quite big, very quickly. The term...
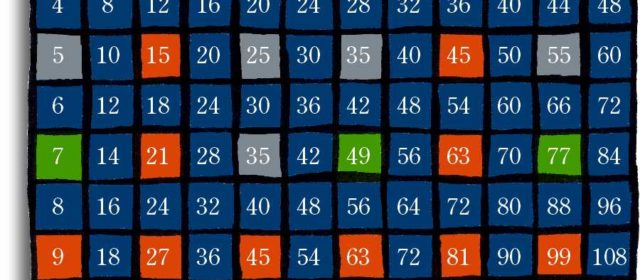
Read MoreJust for fun – this is Emily’s Calculator. All the numbers are colour-coded to their lowest prime factor according to Emily’s colour scheme. Emily doesn’t tackle zero as she interested in counted things that exist in the world. Zero is coloured black as it has a very special status of its own. This is an example of a square of numbers, similar to a small times table, where the highest number in the top-right...
Read MoreEmily invents the threeven numbers as the subset of the odd numbers that all have three as a factor. She knows, intuitively by looking at her counting numbers, that a third of all positive integers are divisible by three with no remainder. She can also see in her numbers that half of these numbers have already been counted in the even numbers. So, to calculate the portion of threeven numbers: And the portion of remaining...
Read MoreThis is the table of 144 numbers that Emily, and millions like her, are drilled on every week at school. The numbers have been colour-coded to their lowest prime factor according to Emily’s scheme. Each number is repeated 2.5 times on average and some numbers appear 6 times. The table only contains the safe, friendly and boring numbers. Here is another table of 144 numbers with each appearing only once. We can see the odd...
Read MoreHere is some Python code that uses Emily’s approach to find all the primes up to a specified number. Set ‘max’ to an integer up to which you want to find prime numbers. Click run. The code returns: a list of primes; the number of primes calculated from each oddish sequence; and the number primes up to ‘max’. The method is similar to the Sieve of Eratosthenes, but it only marks each non-prime once and...
Read More




Recent Comments